Harmonic Distance
Harmonic distance is the total length of the connection between two notes on the lattice, as measured on the solid lines. The more tinkertoy sticks you traverse to get from one note to the other, the greater the harmonic distance.
It’s not the same thing as melodic distance, which is a difference in pitch. Two notes can be far apart in harmonic space, but close together in melodic space, or vice versa. This post has a demonstration.
Each solid line on the lattice is a prime factor — 3, 5 or 7. A simple way to put a number on harmonic distance is to multiply together all the prime factors used in the ratio of the interval. Doesn’t matter if you’re multiplying or dividing by the factor, the distance is the same. Twos don’t count; these are octaves and they don’t add distance on the lattice.
The closest intervals on the lattice are the perfect fifth and perfect fourth. To get these intervals, you multiply or divide the original note by 3. The ratio of the fifth is 3/1, and the ratio of the fourth is 1/3. The harmonic distance is 3, in both cases.
The major seventh, or 7, is a more distant interval. Its formula is x3, x5, or 15/1, so its harmonic distance is 15.
The b2- is the reciprocal of the 7. Its formula is ÷3, ÷5, or 1/15, and it is equally distant. The polarity is opposite, but it’s the same distance away from the center.
There are two other notes at this same distance of 15 — the 6 and the b3. Their ratios are 5/3 and 3/5 respectively. They are reciprocals of each other, and have opposite polarities.
Here is the inner lattice, showing the ratios (without any factors of 2), and harmonic distances instead of the note names. The ratio of an interval defines it completely; it would make perfect sense to name the notes by their ratios alone (it’s been done).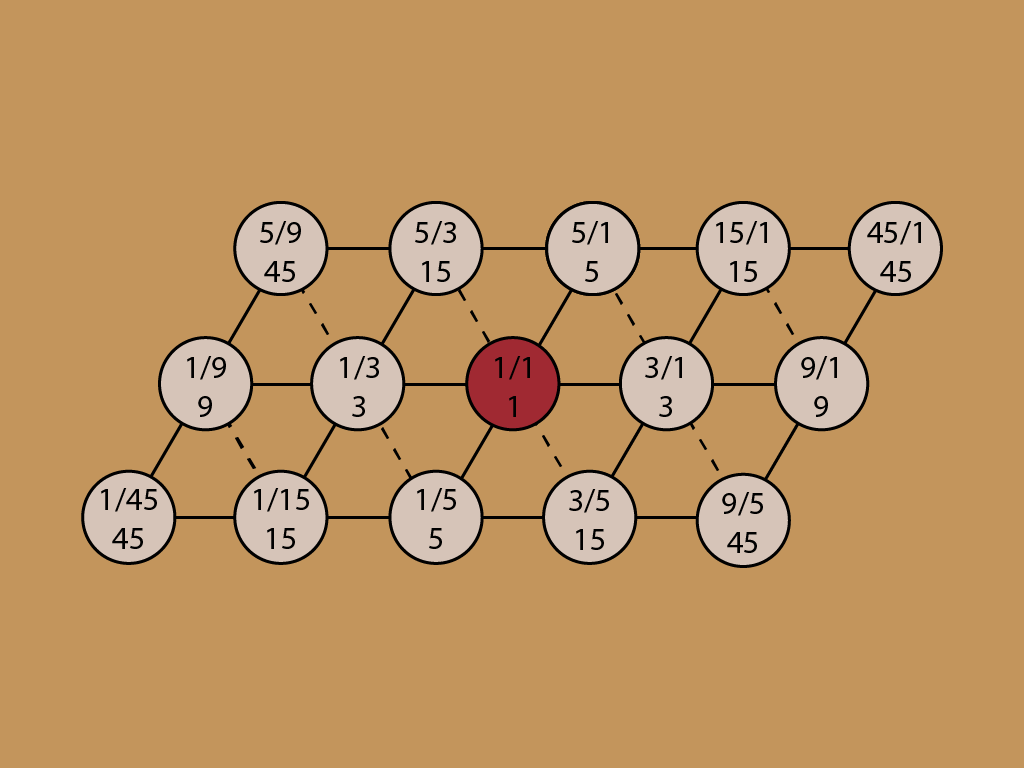
In the consonance experiment from a few posts ago, I played intervals in order of harmonic distance, and sure enough, as they got further out, they got more dissonant. I used the Pythagorean axis (multiples of 3) to keep it simple. Pythagorean tuning is somewhat limited musically; harmonic distance increases so fast that there are very few consonant notes.
On the lattice of thirds and fifths, there are more consonant notes to play with. How would that same experiment sound, when you add in these new intervals?
I’ll stick with the overtonal, Northeast quadrant of the lattice. Every ratio involves multiplication only, so there is no reciprocal energy, and I’m not comparing apples to oranges. My intention is to test only one ingredient of consonance, the harmonic distance. The intervals travel away from the center, and back again. Listen and watch a couple of times, and hear what happens.
I think the pattern holds very nicely. At the very end, the #4+ with its distance of 45, I think the dissonance has lost some of its obnoxiousness. It does appear that as the distance gets big enough, both consonance and dissonance start to weaken. The ear has less to go on, the signal is weaker.
Also note how the other component of consonance, stability/instability, changes as we roam farther out and come home again. All these intervals are stable, since they are all overtonal. This sense of stability gets stronger the closer we are to home, as though the ear is receiving a stronger signal and is more and more sure of itself. I start to clearly hear the stability at the major seventh (15/1), and it quickly gets stronger from there on in.
Next: Mirror Twins
Very good and helpful article.
I’m not sure I go along with the description of reciprocal intervals as “unstable.” As a Hindustani singer, I hear them as consonances — different in quality, perhaps, but no less stable.
Also, I’m not totally on board with some of your conceptualizations of dissonance, which seem to some extent predicated on conceptions of tonality derived from a 12-tone tempered universe, even when you’re talking about just intervals with simple ratios.
For example, I sing the natural 7th against a 1-5 tamboura drone, and it’s a 5:4 over 3:2 — a major third off the perfect fifth — and my ears hear it as totally stable, with no “pull” to resolution at all. I’ll hang out there all day with no need to move elsewhere. I hear it as a “complex consonance,” just as stable as its reciprocal counterpart, the 16:15 minor 2.
Maybe that’s because I approach these relationships as part of a “dronality,” not a “tonality.”
I agree with much of what you say. The 15/8 seventh sounds stable to me too — though more weakly than the fifth or third of which it is composed. Same with the 9/8 major second; it has a wistful sound, but I could hang out there all day. But both of these notes are overtonal, though somewhat distant in harmonic space.
I’m curious about the 16/15 though! To me it sounds weakly unstable. I have a video comparing the 7 and b2- here: https://www.youtube.com/watch?v=Njf5NcZX8Hs
In dronality, do you hear any notes as unresolved? Which ones? Does it depend on context?
My expectations are surely a factor, coming from a Western, tonality-based background. I apologize if I seem dogmatic or prescriptive — Gamelan music and Arabic quarter-tones don’t depend much on these ideas at all! If it sounds good, it is good.